
In light of that, we can utilize the Pythagorean hypothesis, which expresses that if a and b are the legs of a right-angled triangle and c is the hypotenuse (or the longest side), at that point a 2 + b 2 = c 2.

This divides the triangle into two right triangles. The line depicting the height of an isosceles triangle crosses the base so that it cut the base down from the middle. The length of the base is 7 centimetres, so to determine the area you should find the height of the sticker. It does not indicate the height or the area of the sticker, and you need to find the area to realize how a lot of room it will take up on your windshield. The sticker provides the lengths of its sides as 9cm, 9cm and 7cm. It is significantly more typical that we would have the lengths of the base and other sides of an isosceles triangle than it is that we would have the height of the triangle.įor instance, assume you purchase a state park sticker for the windshield of your vehicle that is looking like an isosceles triangle. In any case, we frequently aren't given the height of an isosceles triangle, and we unquestionably need this to apply the formula. Area of Isosceles Triangle (if all sides are given) ½(a 2 b 2 /4) × b where, b base of the isosceles triangle a length of the two equal sides.
#Area of isosceles triangle full#
Interior angles in a quadrilateral sum to a full turn (360). Quadrilaterals have four sides and four angles. We have figured out that finding the area of an isosceles triangle is equivalent to finding the area of any triangle - we utilize the equation for the area of a triangle, A = (1/2) b x h. Base interior angles in an isosceles triangle are equal. Determination of Height of Isosceles Triangle We determined that the area of an isosceles triangle with base 10 centimetres and height 12 centimetres is 60 square centimetres. To calculate the area of an isosceles triangle, we use the below formula:įor instance, consider an isosceles triangle with a base of 10 centimetres and a height of 12 centimetres, we would put the values, b = 10 and h = 12 into the formula to determine the area, like this: Until now, we have made ourselves familiar with different parts of an isosceles triangle, let's concentrate on the whole part. The length from the centre point of the base to the vertex is known as the height of the triangle. The points on which the sides of the triangle meet are called vertices. In an isosceles triangle, we consider the two equivalent sides as the legs of the triangle, and we consider the opposite side as the triangle base. Be that as it may, before we look into the formula, we should initially acquaint ourselves with the various parts of an isosceles triangle. The particular formula is applicable for all triangles. An isosceles triangle has only two sides of equivalent length, similar to the one depicted below.Īs a rule, with regards to a triangle, we have a decent equation that we can use to determine the area of the triangle. Fundamentals of Isosceles Triangle It is helpful to characterize an isosceles triangle to determine the area of an isosceles triangle.
#Area of isosceles triangle how to#
We'll additionally see how to find the height of an isosceles triangle given the lengths of its sides since this is required to determine the area of a triangle.
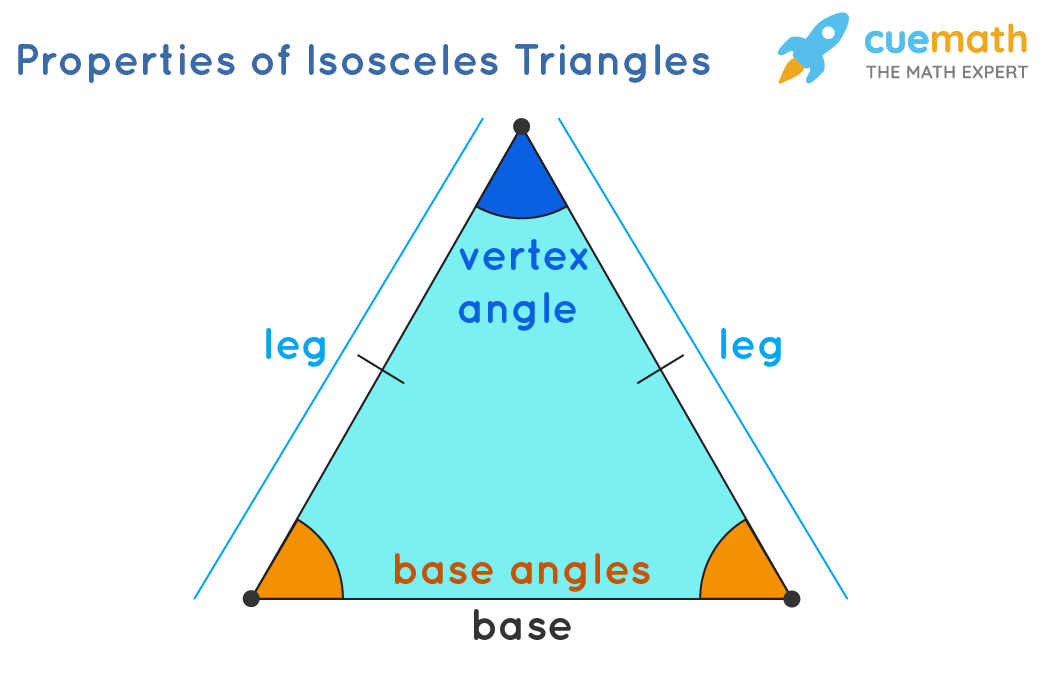

Here we have three formulas to find the area of a triangle, based on the given parameters.Īrea = \(\frac\) Such special properties of the isosceles triangle help us to calculate its area as well as its altitude with the help of the isosceles triangle formulas.Īrea of an Isosceles Triangle: It is the space occupied by the triangle. Thus, in an isosceles triangle, the altitude is perpendicular from the vertex which is common to the equal sides. What Are the Isosceles Triangles Formulas?Īn isosceles triangle has two sides of equal length and two equal sides join at the same angle to the base i.e. The two important formulas for isosceles triangles are the area of a triangle and the perimeter of a triangle. Various formulas for isosceles triangles are explained below. The two angles opposite to the equal sides are equal and are always acute. In this figure, a- Measure of the equal sides of an isosceles triangle. In geometry, an isosceles triangle is a triangle having two sides of equal length. An isosceles triangle is a triangle with 2 sides of equal length and 2 equal internal angles adjacent to each equal sides.


 0 kommentar(er)
0 kommentar(er)
